
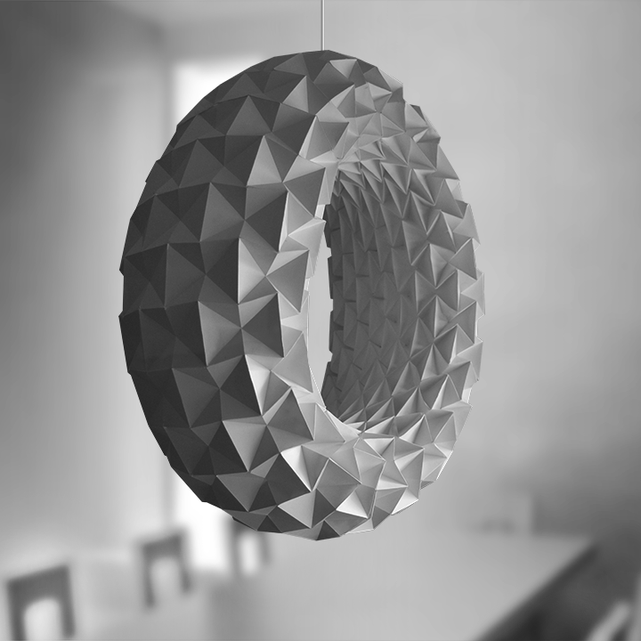

Torus
2015
Diameter 24"
Hi-tec Kozo, plastic, LED
Torus is folded from one single sheet of uncut paper. Gauss’s Theorema Egregium states that the Gaussian curvature of a surface doesn’t change if one bends the surface without stretching it. Therefore, the isometric embedding from a flat square or rectangle to a torus is impossible. The famous Hévéa Torus is the first computerized visualization of Nash Problem: isometric embedding of a flat square to a torus of C1 continuity without cutting and stretching. Interestingly, the solution presented in Hévéa Torus uses fractal hierarchy of corrugations that are similar to pleats in fabric and folds in origami. In my Torus, isometric embedding of a flat rectangle to a torus of C0 continuity is obtained by using periodic waterbomb tessellation.
To learn more about using Waterbomb to create a torus, check out the following paper:
Wu, J. (2020). Geometric Strategies in Creating Origami-Inspired Light Art. Sriraman, B. (Eds.), Handbook of the Mathematics of the Arts and Sciences, Cham, Switzerland: Springer.
Awards:
JMM Math Art Exhibition, Mathematical Association of America, Best textile, sculpture, or other medium Award.
